函式名
 特殊三角函式
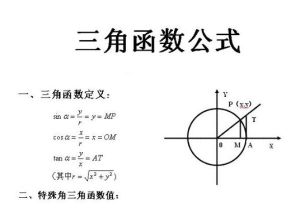
特殊三角函式在平面直角坐標系xOy中,從點O引出一條射線OP,設鏇轉角為θ,設OP=r,P點的坐標為(x,y)有
正弦函式sinθ=y/r
餘弦函式cosθ=x/r
正切函式tanθ=y/x
餘切函式cotθ=x/y
正割函式secθ=r/x
餘割函式cscθ=r/y
正弦(sin):角α的對邊比上斜邊
餘弦(cos):角α的鄰邊比上斜邊
正切(tan):角α的對邊比上鄰邊
餘切(cot):角α的鄰邊比上對邊
正割(sec):角α的斜邊比上鄰邊
餘割(csc):角α的斜邊比上對邊
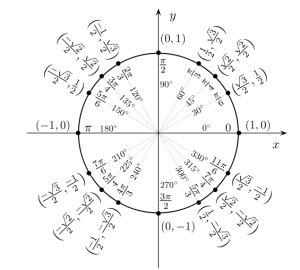
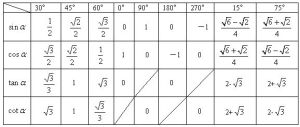
特殊角函式
角度a:0°、30°、45°、60°、90°、120°、180°sina01/2√2/2√3/21√3/20
cosa1√3/2√2/21/20-1/2-1
tana0√3/31√3無限大-√30
cota/√31√3/30-√3/3/
倒數關係
tanα·cotα=1sinα·cscα=1
cosα·secα=1
商的關係
tanα=sinα/cosαcotα=cosα/sinα
平方關係
 特殊三角函式表
特殊三角函式表1+(tanα)^2=(secα)^2
1+(cotα)^2=(cscα)^2
以下關係,函式名不變,符號看象限
sin(2kπ+α)=sinα
cos(2kπ+α)=cosα
tan(2kπ+α)=tanα
cot(2kπ+α)=cotα
sin(π+α)=-sinα
cos(π+α)=-cosα
tan(π+α)=tanα
cot(π+α)=cotα
sin(π-α)=sinα
cos(π-α)=-cosα
tan(π-α)=-tanα
cot(π-α)=-cotα
sin(2π-α)=-sinα
cos(2π-α)=cosα
tan(2π-α)=-tanα
cot(2π-α)=-cotα
以下關係,奇變偶不變,符號看象限
sin(90°-α)=cosα
cos(90°-α)=sinα
tan(90°-α)=cotα
cot(90°-α)=tanα
sin(90°+α)=cosα
cos(90°+α)=sinα
tan(90°+α)=-cotα
cot(90°+α)=-tanα
sin(270°-α)=-cosα
cos(270°-α)=-sinα
tan(270°-α)=cotα
cot(270°-α)=tanα
sin(270°+α)=-cosα
cos(270°+α)=sinα
tan(270°+α)=-cotα
cot(270°+α)=-tanα
積化和差公式
sinα·cosβ=(1/2)*[sin(α+β)+sin(α-β)]cosα·sinβ=(1/2)*[sin(α+β)-sin(α-β)]
cosα·cosβ=(1/2)*[cos(α+β)+cos(α-β)]
sinα·sinβ=(1/2)*[cos(α+β)-cos(α-β)]
和差化積公式
sinα+sinβ=2*[sin(α+β)/2]*[cos(α-β)/2]sinα-sinβ=2*[cos(α+β)/2]*[sin(α-β)/2]
cosα+cosβ=2*[cos(α+β)/2]*[cos(α-β)/2]
cosα-cosβ=-22*[sin(α+β)/2]*[sin(α-β)/2]
三倍角公式
sin(3α)=3sinα-4sin^3α=4sinα·sin(60°+α)sin(60°-α)cos(3α)=4cos^3α-3cosα=4cosα·cos(60°+α)cos(60°-α)
tan(3α)=(3tanα-tan^3α)/(1-3tan^2α)=tanαtan(π/3+α)tan(π/3-α)
兩角和差公式
sin(α+β)=sinαcosβ+cosαsinβsin(α-β)=sinαcosβ-cosαsinβ
cos(α+β)=cosαcosβ-sinαsinβ
cos(α-β)=cosαcosβ+sinαsinβ
tan(α+β)==(tanα+tanβ)/(1-tanα·tanβ)
tan(α-β)=(tanα-tanβ)/(1+tanα·tanβ)