解析函式項級數
正文
由解析函式組成的級數。在實分析中，可導函式的一致收斂級數不一定可導。例如由外爾斯特拉斯定理知道,在【α,b】上連續的任何函式可表示為一致收斂的多項式級數。在複分析中有不同的結果：一致收斂的解析函式項級數是解析函式。設ƒn(z)(n=1,2,…)是在區域D內連續的函式。如果對任何緊集K嶅D以及任何ε>0,存在著正整數N=N(K,ε)，使得對n≥N及任何z∈K,
,則稱級數
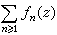（簡寫為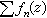
）在D內任何緊集上一致收斂。如果對任何緊集K嶅D，級數
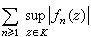收斂，則稱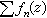在D內任何緊集上正規收斂。正規收斂性在套用中是常見的；顯然，如果 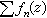在D內任何緊集上正規收斂，那么它在這種集上一致收斂。 套用柯西公式（見柯西積分定理），K.外爾斯特拉斯證明了下列定理：設ƒn(z)(n=1,2,…)在區域 D 內解析,如果
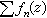在D內任何緊集上一致收斂，那么它的和ƒ(z)在D內解析，而且在D內，
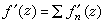，此式右邊的級數在D內任何緊集上一致收斂。如果 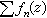在D內任何緊集上正規收斂，那么級數
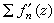在D內任何緊集上也正規收斂。 形如
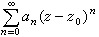(簡記為
,式中αn和z0為複數)的級數是一種特殊的解析函式項級數,稱為冪級數。 對於這種級數有下列阿貝爾引理：設
在z1≠z0收斂。則對滿足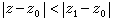
的任何z，級數絕對收斂。 由這引理出發，可以證明任何冪級數
屬於下列三種情況之一。 ① 存在著有限正數R;級數在圓盤|z-z0|<R內絕對收斂而且在這圓盤內任何緊集上正規收斂;當|z-z0|>R時，級數發散。這時R稱為級數的收斂半徑，|z-z0|<R稱為收斂圓盤，|z-z0|=R 稱為收斂圓周。
② 對任何z≠z0，級數發散；這時稱級數的收斂半徑為0。
③ 對任何z，級數收斂，從而在任何緊集上正規收斂；這時稱級數的收斂半徑為+∞。
由外爾斯特拉斯定理，在第一種情況下，冪級數在收斂圓盤內解析，並且可逐項求導數；在第三種情況下，冪級數表示一整函式(即在整個有限複平面解析的函式)，並且可在有限複平面內逐項求導數。
在第一種情況下，冪級數在其收斂圓上的點可能收斂，也可能發散。例如
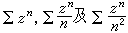的收斂半徑都是1,而在收斂圓周上，第三個級數處處收斂；第一個級數處處發散；第二個級數在－1收斂,在1發散(可證明它在收斂圓周上除去1外處處收斂)。對於在圓周上某些點收斂的冪級數，有下列阿貝爾－施托爾茨定理：設冪級數有收斂半徑R(0<R<+∞)，並且它在收斂圓周上一點z*收斂。作以z*為頂點、以z0及z*的聯線為平分角線，並且角度小於π的角。那么當z在收斂圓盤內且在這角域內趨近於z*時，有 
。
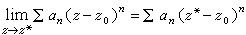的收斂半徑R可以用下列柯西-阿達馬公式求出：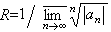 
；
